Lesson Plan and Lesson Note
Lesson Note on Mensuration
Theme: Mensuration
Topic: Areas of Plane Shapes
Sub Topic: Area of Triangles
Date: xx/xx/xxxx
Class: J.S.S.3
Duration: 35 Minutes
No of Learners: 30
Learning Objectives:
By the end of the lesson learners should be able to:Understand the concept of area.
Understand the given sides/angle of a Triangle, and derived the concept of the area of a Triangle.
State the formulae of the area of a triangle under a specific condition(s).
- Solve problems in the area of a triangle.
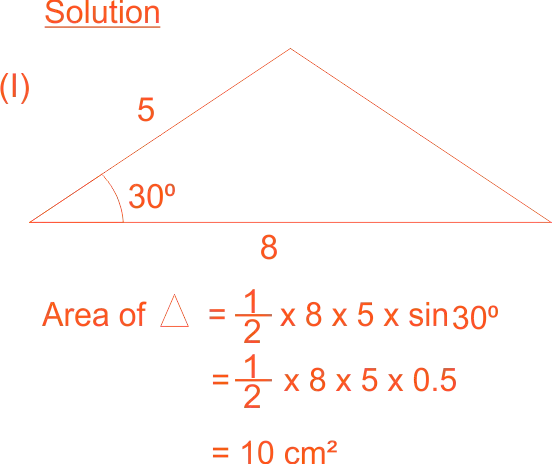
- Calculate the area of a triangle with a base of 8cm and a height of 6cm.
SOLUTION
Area of Triangle = lenght x bright
= 1/2 x 8 x 6
= 24 cm sq
- Calculate the area of a triangle with a base of 8cm and a height of 6cm.
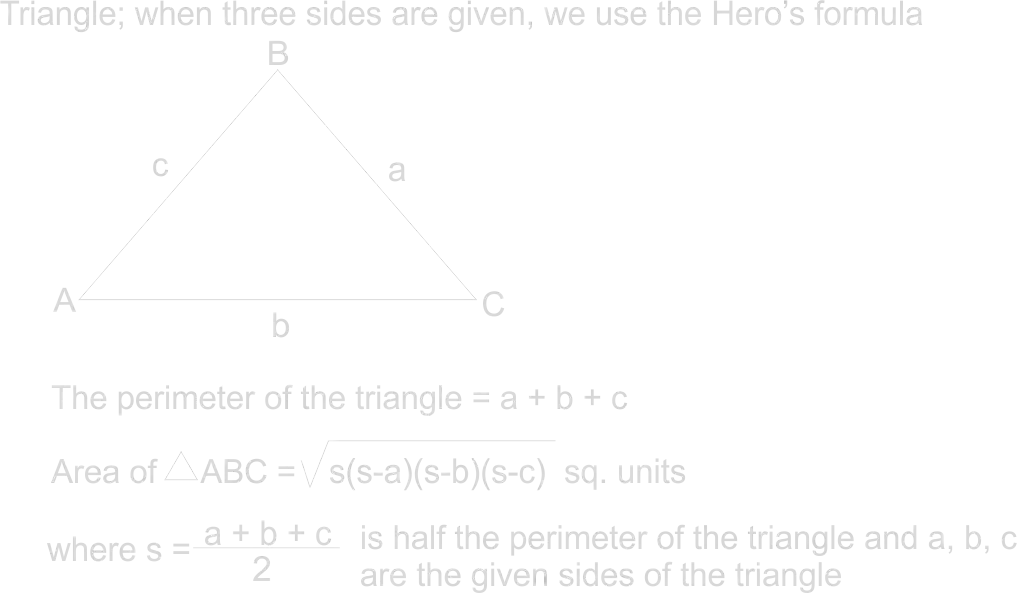
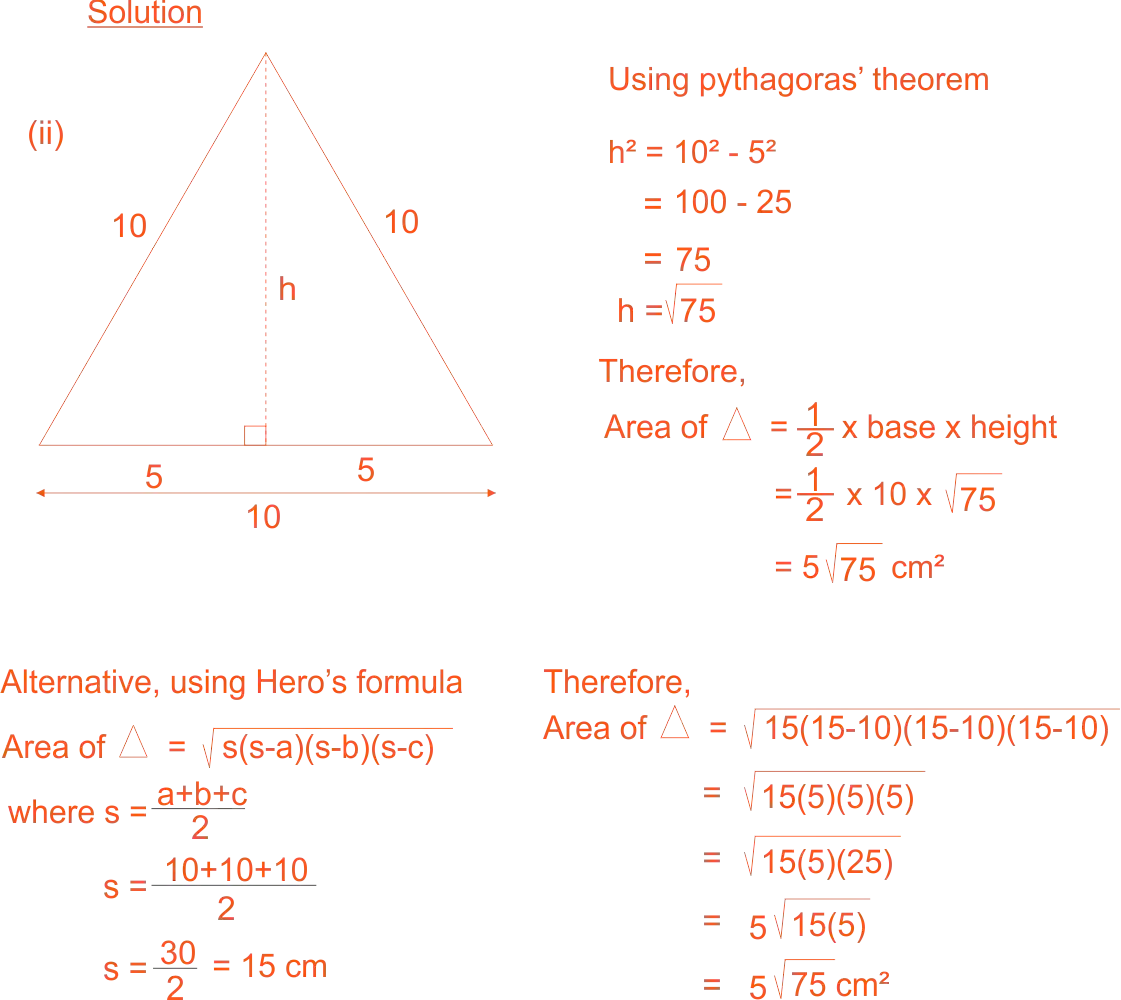
- Find the area of a triangle whose dimensions are 3cm, 4cm, and 5cm.
SOLUTION
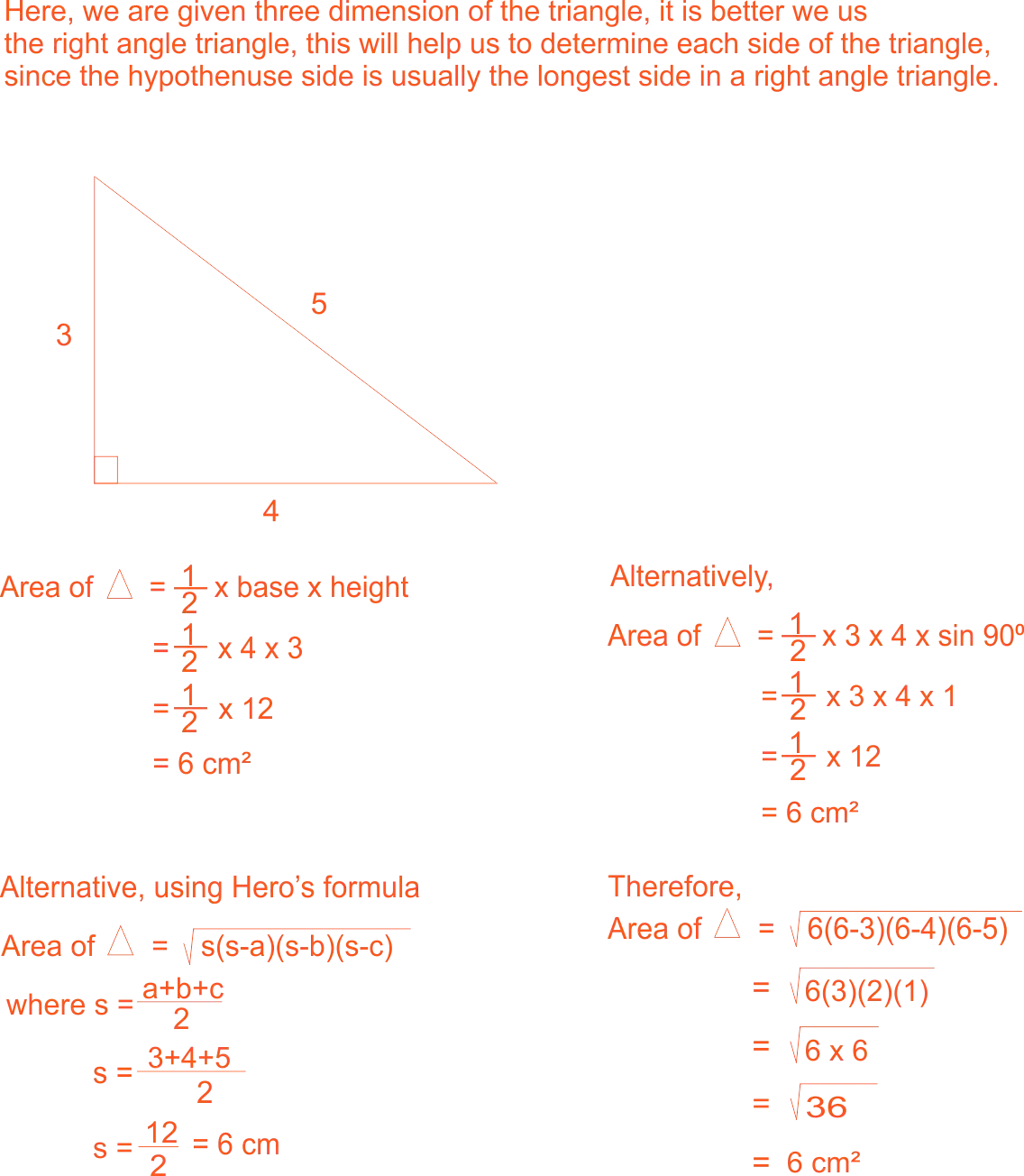
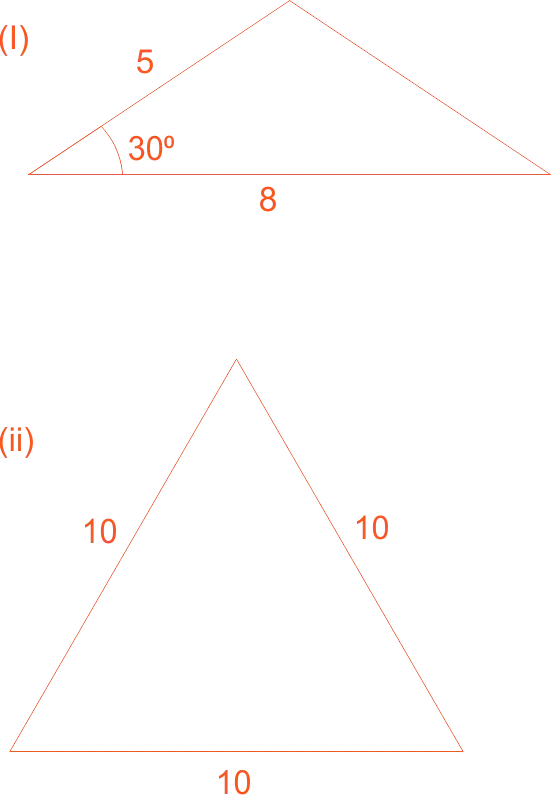
- Calculate the area of the diagrams below.
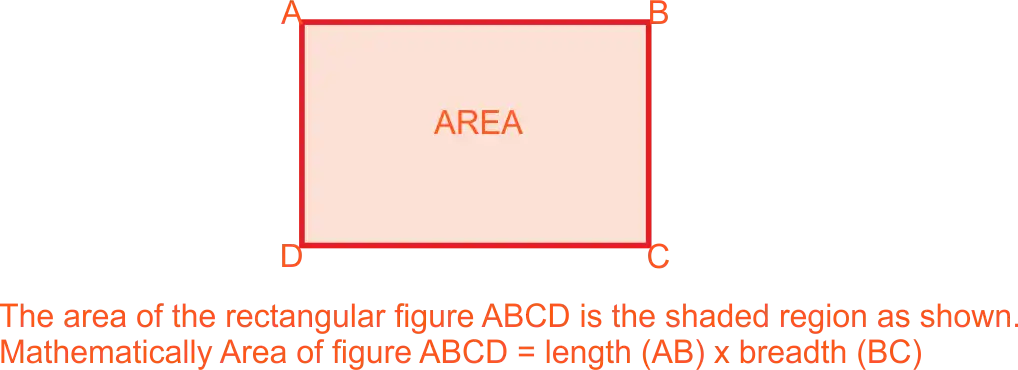
The area of an object can be taken to be an enclosed region bounded by either straight lines and/or curve or arcs. The area of an object is the space occupied by that object.
Mathematically, the concept of area is usually conceived as length multiplied by breadth.

Rationale:
There are many authentic and real-life reasons why we would need to calculate the area of various shapes. For instance, suppose you are looking to sod your lawn; you would need to know the area of your lawn to purchase enough sod. Or, you may wish to lay carpet in your living room, halls, and bedrooms. Again, you need to calculate the area to determine how much carpeting to purchase for the various sizes of your rooms. Knowing the formulas to calculate areas will help you determine the areas of the rooms.
Prerequisite/ Previous knowledge:
Measurements, scale drawing, ratios, conversions from one unit to another, sets.
Teaching/ Learning Materials:
Mathematical set, real objects, worksheets, pencils, rulers, 2D dimensional shapes.
Reference Materials:
New General Mathematics for Junior Secondary Schools Books 3 By Pearson, Advance level Mathematics, Ordinary Level Mathematics.
Lesson Development:
| STAGE | TEACHER'S ACTIVITY | LEARNER'S ACTIVITY | LEARNING POINTS |
|---|---|---|---|
| INTRODUCTION full class session (5mins) |
The teacher provides learners with several different objects, figures/shapes and asks learners to:
|
Learners respond to the teacher's questions:
|
Learner’s entry points. |
| STEP 1 20mins. Development and Grouping |
How would you describe the area of the rectangular A4 papers? The teacher explains to learners that the concept of area is usually conceived as length multiplied by the breadth and that the area of an object can be taken to be an enclosed region bounded by either straight lines and/or curves or arcs. |
Students respond to the teacher's question.
|
Developing the ideal of the concept area. |
| STEP 2 10mins. Area of a triangle. |
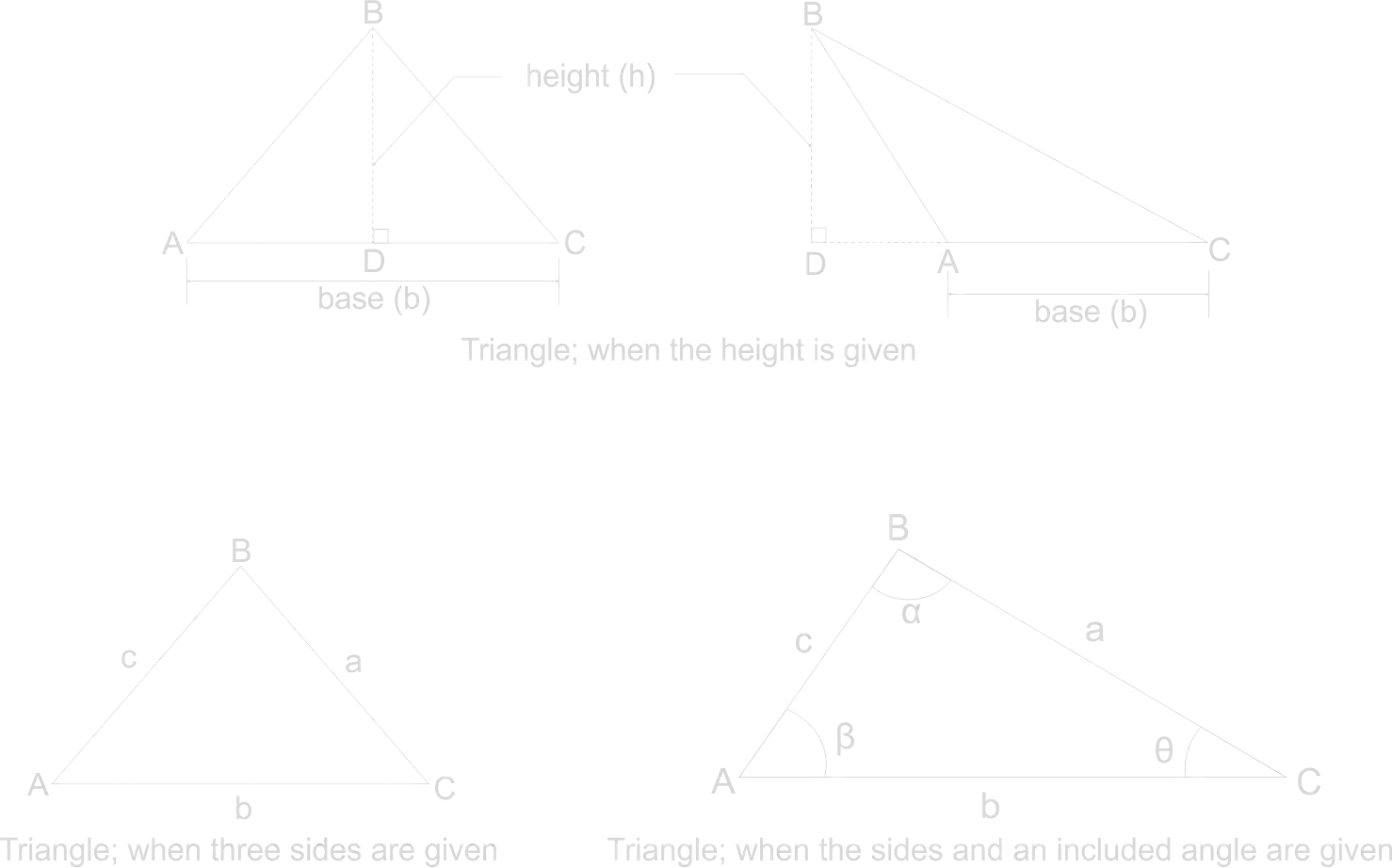
The teacher asks learners to study the triangles in the learner's activity and understand how the sides and angles are named. | 
Learners observed that each angle is named with upper case letters while the side facing each angle is named with lower case letters of the angles respectively, learners also observed how the height and base of a rectangle are determined. |
Developing and improving the idea of a triangle. |
The teacher explains the area of a triangle to learners.
The teacher guides the learners to derive the formula of a triangles
|



|
Identify similar objects by comparing corresponding sides and angles. | |
| EVALUATION 5mins |
The teacher asks the students questions.
|
Learners respond to teacher's questions | Ask the learners questions to assess the achievement of the set objectives. |
| ASSIGNMENT | Teacher gives learners take a home. | 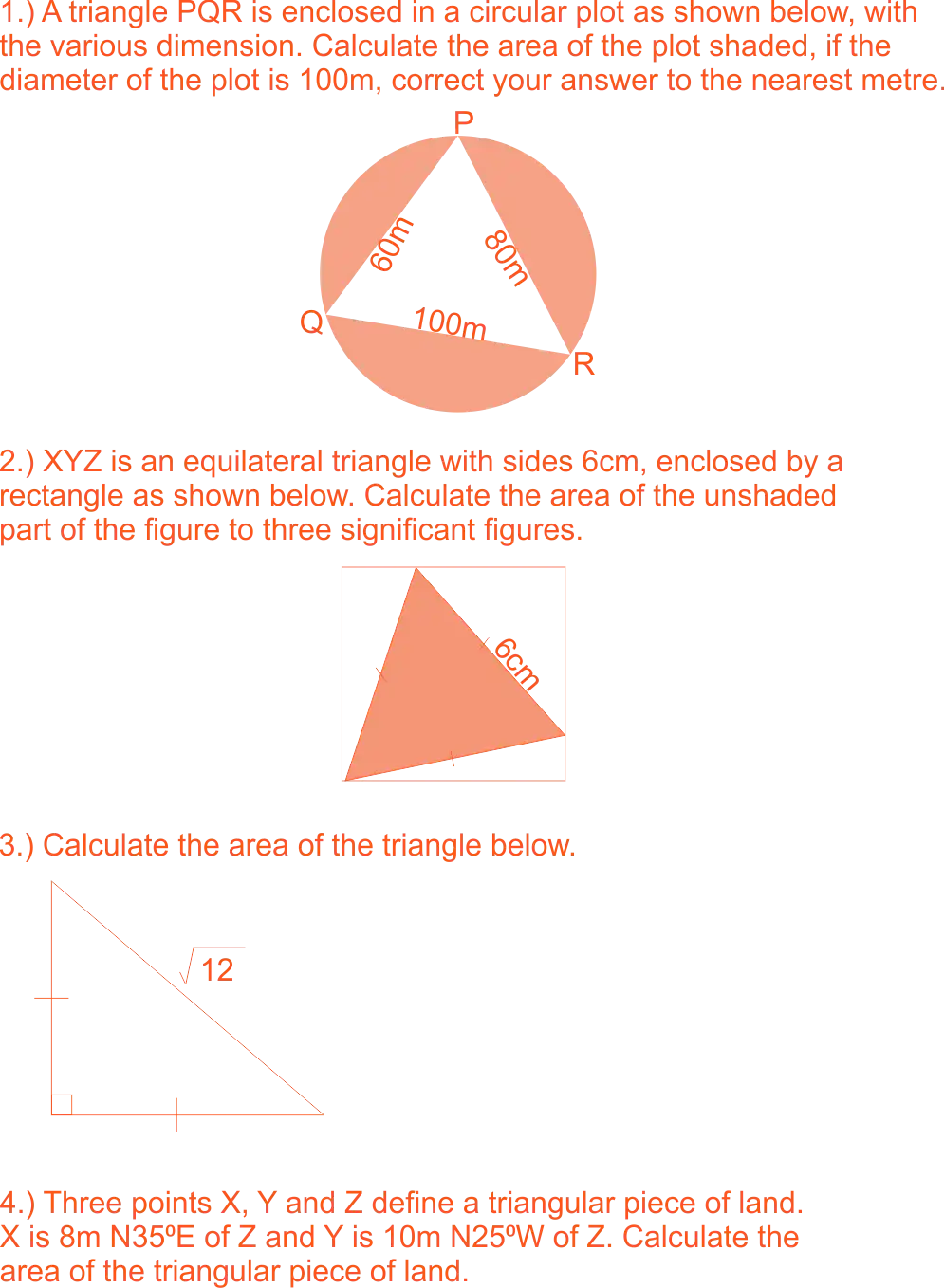
| Improving their level of understanding of the Area of a Triangle. |
| CONCLUSION 5mins |
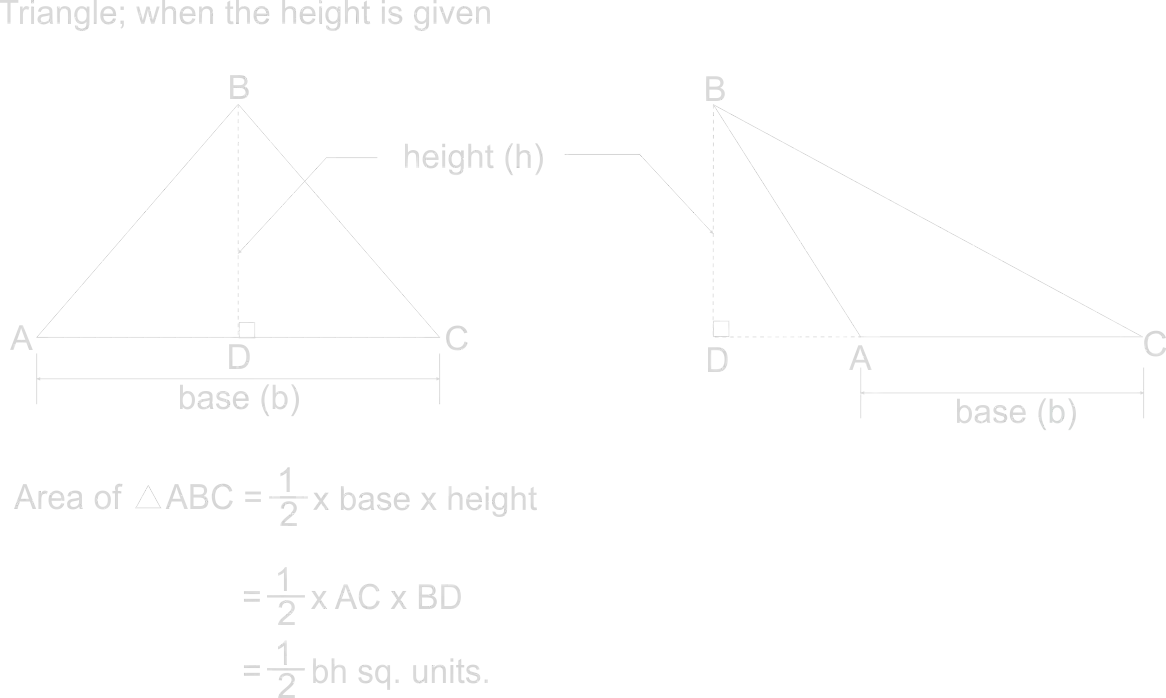
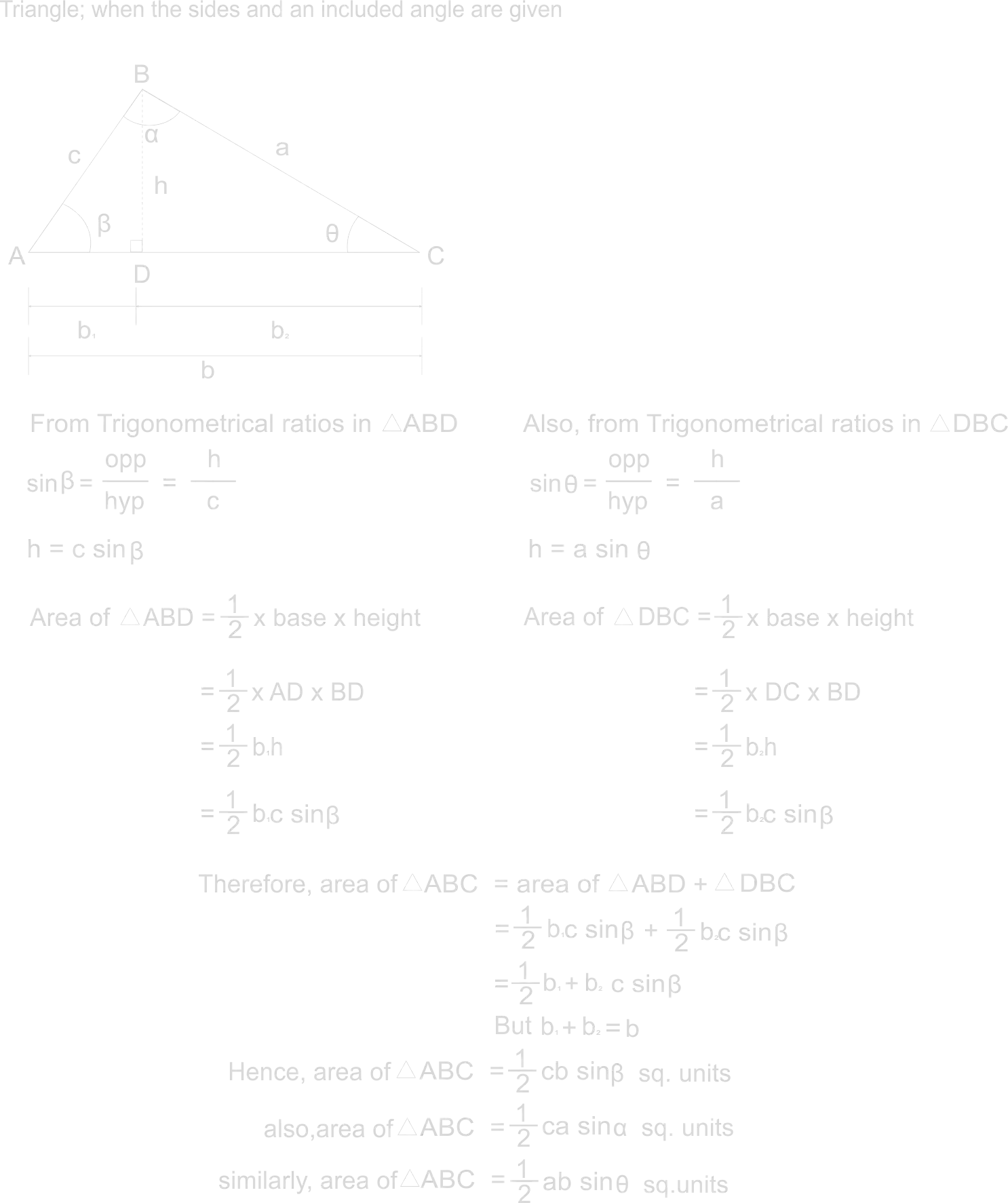
How would you find the area of a triangle, when;
|
The area of the triangle is;



|
Improving their level of understanding of the Area of a triangle. |