Lesson Plan Sample
Lesson Note on Arithmatic Sequences
Theme: Sequences and Series
Topic: Sequences and Series II
Sub Topic: Arithmatic Sequences
Date: dd/mm/yyyy
Class: Basic 6
Duration: 35 Minutes
No of Learners: 30
Learning Objectives:
By the end of the lesson learners should be able to:Derive the general formula of a particular arithmetic sequence (progression) A.P
- 1, 2, 3, 4, 5, ....
- 5, 10, 20, 40, 80, ....
- -3, -1, 1, 3, 5, ...
- Term = T
- first term = a or T1
- constant difference b/w any two consecutive terms = d
- nth term = Tn
Apply the general formula to solve problems.
- Find the 9th term of the sequence 6, 11, 16, 21, 26, ...
- The 10th term of an A.P is 47, the 4th term is 17. Write down the first 3 terms of the sequence.
- T1 => a = 6
d = T2 - T1 or T3 - T2 or T4 - T3 ...
d = 11 - 6 = 5
T9 = ?
Since Tn = a + (n - 1)d
:. T9 = 6 + (9 -1)5
T9 = 6 + 40
T9 = 46 - T10 = a + (10 - 1)d => a + 9d = 47
T4 = a + (4 - 1)d => a + 3d = 17
These are now two simultaneous equations
a + 9d = 47 -----------(i)
a + 3d = 17 -----------(ii)
By eliminating, 6d = 30:. d = 5
From (ii) a + 3d = 17; substituting for d in (ii):. d = 5
:. The first term of the sequence T1 = 2
The second term of the sequence T2 = a + (2 - 1)d
T2 = 2 + (1)5 => 2 + 5 => 7
The third term of the sequence T3 = a + (3 - 1)d
T3 = 2 + (2)5 => 2 + 10 => 12
:. The first three terms of the sequence are 2, 7, 12
Example of sequence;
In an Arithmetic Progression, A.P, it is conventional to denote the:
From the example of the sequence above; 1, 2, 3, 4, 5,...
T1 = 1 = a
T2 = 2 = a + d => a + (2 - 1)d
T3 = 3 = a + d + d => a + 2d => a + (3 - 1)d
T4 = 4 = a + d + d + d => a + 3d => a + (4 - 1)d
T5 = 5 = a + d + d + d + d => a + 4d => a + (5 - 1)d
Following this pattern,
T6 = a + (6 - 1)d and
T7 = a + (7 - 1)d and so on.
Therefore, nth term Tn = a + (n - 1)d
Rationale:
Mathematical skills to explore number patterns are applied to our daily life such as in the design of clothes and ornaments. Also, the idea of the sequence is applied to our daily life such as in calculating simple and compound interest, and accumulated amounts in banks.
Prerequisite/ Previous knowledge:
- Defining a sequence and an arithmetic sequence
- Skills of generalisation and prediction
- Simple number patterns
Learning Materials:
Matchsticks, worksheet on manila paper
Reference Materials:
- Cambridge University Press, 1994, ‘School Mathematics of East Africa Book 1’, Cambridge University Press, second edition,
- General Mathematics for Senior Secondary 2.
Lesson Development:
| STAGE | TEACHER'S ACTIVITY | LEARNER'S ACTIVITY | LEARNING POINTS |
|---|---|---|---|
| INTRODUCTION full class session (5mins) |
The teacher writes the following sequences on a whiteboard and the learners write them down in their notebooks. 4, 7, 10, 13, 16, ... 3, 8, 13, 18, ...
|
Learners respond to teacher questions
|
Learner’s entry points. Confirming the previous knowledge. |
| STEP 1 12 mins. Development |
|
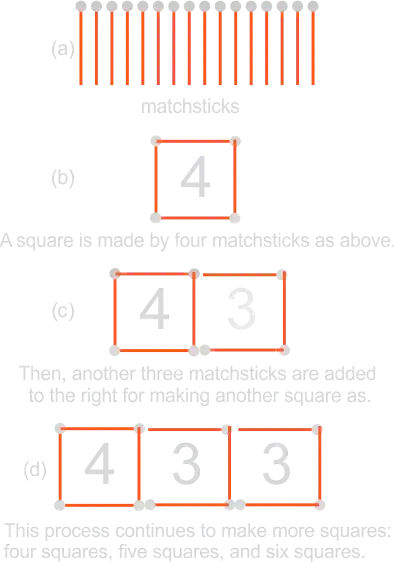 Activity of sequence using matche stickes
Activity of sequence using matche stickes
|
Identification of the patterns |
ACTIVITY
Learners carry out the following activities presented in printed paper, manila sheets or simply written on a whiteboard by a teacher.
The learners complete the table and identify the pattern of getting the number of matchsticks used to make the squares (Minds-on activity).
Learners count the number of matchsticks required in the cases of four, five and six squares. Then, complete the following table.| No. of squares formed | No. of matchsticks used | The way of calculating no. of matchsticks used | The way of calculating no. of matchsticks used |
|---|---|---|---|
| 1 | 4 | 4 | 4 |
| 2 | 7 | 4 + 3 | 4 + d |
| 3 | 10 | 4 + 3 + 3 | 4 + 2d |
| 4 | |||
| 5 | |||
| 6 | |||
| STEP 2 10 mins. |
The teacher asks learners to find the number of matchsticks needed to form:
|
Through group discussion, the learners get the number of matchsticks used to make 30 and 100 squares, using the patterns in the table. (Minds-on activity: bridging between activity and concept) | The number of times the common difference occurs is (n–1) in the nth term. |
| The learners derive the general form of getting the nth term of this particular arithmetic sequence. (Minds-on activity: bridging between activity and concept). The learners report and explain their results to the other learners on a whiteboard. |
Induction of a general form: Tn = 4 + (n – 1)d where d = 3 | ||
| STEP 3 Group Work (8 mins) |
The teacher asks the learners to get the 20th term of the arithmetic sequence given at the beginning of the lesson and review the predictions made earlier. | 4, 7, 10, 13, 16, ... The learners explain how to get the 20th term. a = 4 d = 7 - 4 = 3 T20 = ? Tn = a + (n - 1)d T20 = 4 + (20 - 1)3 T20 = 4 + (19)3 T20 = 4 + 57 T20 = 61 |
Application of the general form: substituting a number |
| EVALUATION 5 mins |
The teacher asks the students questions.
|
Learners attempted teacher's questions.
|
Asking the learners questions to assess the achievement of the set objectives. |
| CONCLUSION 2 mins |
Teachers wrap up from the learners' observations. | The learners derive the general form for getting the nth term of the other arithmetic sequence given at the beginning of the lesson. | Taking the initial term as three and common difference as five. |
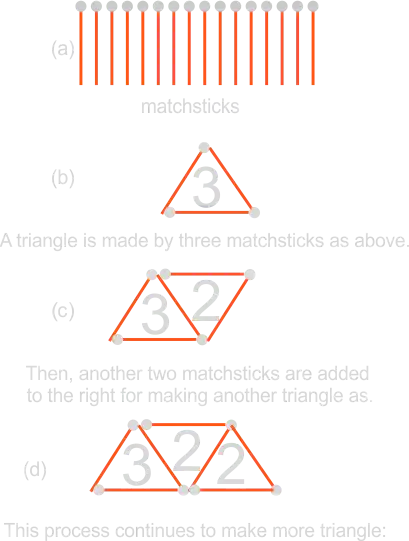
| ASSIGNMENT | Triangles are made following the pattern below. Draw the next two patterns for getting the number of matchsticks to make 4 and 5 triangles.
|
Learners solve other problems. | Improving their level of understanding of AP. |