Lesson Note and Plan on Mathematics

Addition


Subject: Mathematics
Theme: Basic operations
Topic: Addition
Date: dd/mm/yyyy
Class: Basic 2
Duration: 35 Minutes
No of Learners: 30

Learning Objectives:

By the end of the lesson learners should be able to:
1) add 2-digit numbers without exchanging or renaming
  • addition 1
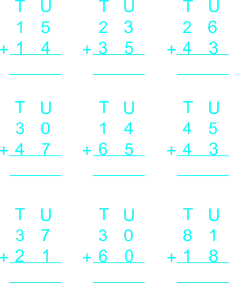
2) add 3-digit numbers without exchanging or renaming
  • addition 2

3) add 2-digit numbers with exchanging or renaming
  • addition 3

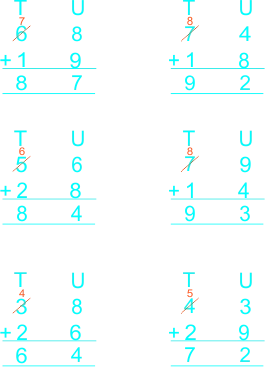
4) add 3 numbers taking two at a time.
  • addition 4

5) real life application (Word problems)
(i) The sum of twenty-one and fourteen is _____.
(ii) Add fifteen pencils, twelve pencils and eleven pencils altogether.


Rationale:

Mathematics is all about sharpening the problem-solving skills and critical thinking. Missing numbers or finding the missing values are a big part of helping children build problem-solving skills. Further, finding the missing numbers is a fun activity for the children to identify the patterns and even learn how to count. This skill can be used in various scenarios like balancing a checkbook, solving word problems, and completing calculations. The activity of finding missing counting numbers can help your little one to develop mental skills and enhance their fine motor practice of learning. It also helps kids to understand important mathematical operations like addition, subtraction, multiplication, and division.

Prerequisite/Previous knowledge:

Learners have been taught addition of 2-digits numbers


Learning Materials:

1) Number Beads
2) Bean seed
3) Cards, etc
4) Charts on addition of 3-digit numbers without renaming, etc
5) Counters such as, sticks, bottle tops.
6) Addition cards.

Reference Materials:

1) New Method Mathematics for Primary Schools 1. C. F. Oredugba, R. Ohuche, G. Salahu et al
2) Understanding Maths Book 1 African First Pub. Ltd. Marian N. Daud-Osuaght
3) New Approach to Quantitative Reasoning Bk. 1 Rasmed T.A.O. Olayiwola.







Lesson Development:
STAGE TEACHER'S ACTIVITY LEARNER'S ACTIVITY LEARNING POINTS
INTRODUCTION
full class session
(3 mins) 		The teacher begins the day's lesson by checking learners' textbook/notebook and writing materials, thereafter revises addition of 2-digit numbers without exchanging or renaming.


The teacher reminded the learners the horizontal method, "FINDING THE TEN (splitting the numbers into tens and unit)".
The teacher tells learners that "The Tens means bundles of 10 and that all 2-digits numbers (10 to 99, 99 is 9 bundles of 10 counters and 9 counters) stands for Tens, its short form is written as T, the Units means 1-digit number (0 to 9) stands for it, its short form is written as U.

E.g 23 + 35
20 + 3 + 30 + 5
Take all the Tens to one side and all the Unit to the other side
20 + 30 + 3 + 5
Add all the Tens together and all the Units together
50 + 8 = 58

The teacher also reminded the learners of the vertical method "PLACE VALUE to split the numbers into Tens and Unit"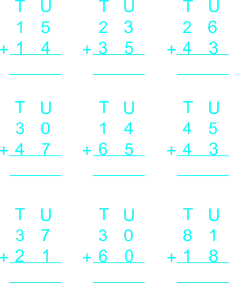
  • addition 5
 Learners expected respond
HORIZONTAL METHOD
(EXPANDED FORM)
1) 15 + 14
10 + 5 + 10 + 4
10 + 10 + 5 + 4
20 + 9 = 29

2) 23 + 35
20 + 3 + 30 + 5
20 + 30 + 3 + 5
50 + 8 = 58

3) 26 + 43
20 + 6 + 40 + 3
20 + 40 + 6 + 3
60 + 9 = 69

4) 30 + 47
30 + 40 + 7
70 + 7 = 77

5) 14 + 65
10 + 4 + 60 + 5
10 + 60 + 4 + 5
70 + 9 = 79

6) 45 + 43
40 + 5 + 40 + 3
40 + 40 + 5 + 3
80 + 8 = 88

7) 37 + 21
30 + 7 + 20 + 1
30 + 20 + 7 + 1
50 + 8 = 58

8) 30 + 60 = 90

9) 81 + 18
80 + 1 + 10 + 8
80 + 10 + 1 + 8
90 + 9 = 99


VERTICAL METHOD
  • addition 6
 Warming up and revising previous lesson addition of 2-digit numbers
DEVELOPMENT
Step 1.
Group Work (5 mins)		 The teacher guides the learners to form four groups and asks them to choose their leaders and secretaries. Learners choose their group leaders and secretaries. Inculcating leadership skills, competitive spirit, cooperation, teamwork and a sense of responsibility among learners.
ACTIVITY 2
Step 3.
Group Work (5 mins)
Review on counting 1-200.		 The teacher review counting 1-200 with the pupils
Add 1 counter to 100 counters represents 101 counters

i.e. 101 counters = 100 counters + 1 counter,

125 counters = 100 counters + 20 counters + 5 counters, e.t.c,

This implies that

123 = 100 + 20 + 3
= 1 Hundred + 2 Tens + 3 Units

251 = 200 + 50 + 1
= 2 Hundreds + 5 Tens + 1 Unit

435 = 400 + 30 + 5
= 4 Hundreds + 3 Tens + 5 Units

The teacher tells learners that the Hundreds, Tens and Units in short form is written as H T U.
The H means bundles of 100; T means bundles of 10; and U means nunmbers from 0-9 		Learners recap on piles build up to correspond with the number given with layout bottle tops in rows and columns of ten up to 100 to counted from one to two hundred (1-200)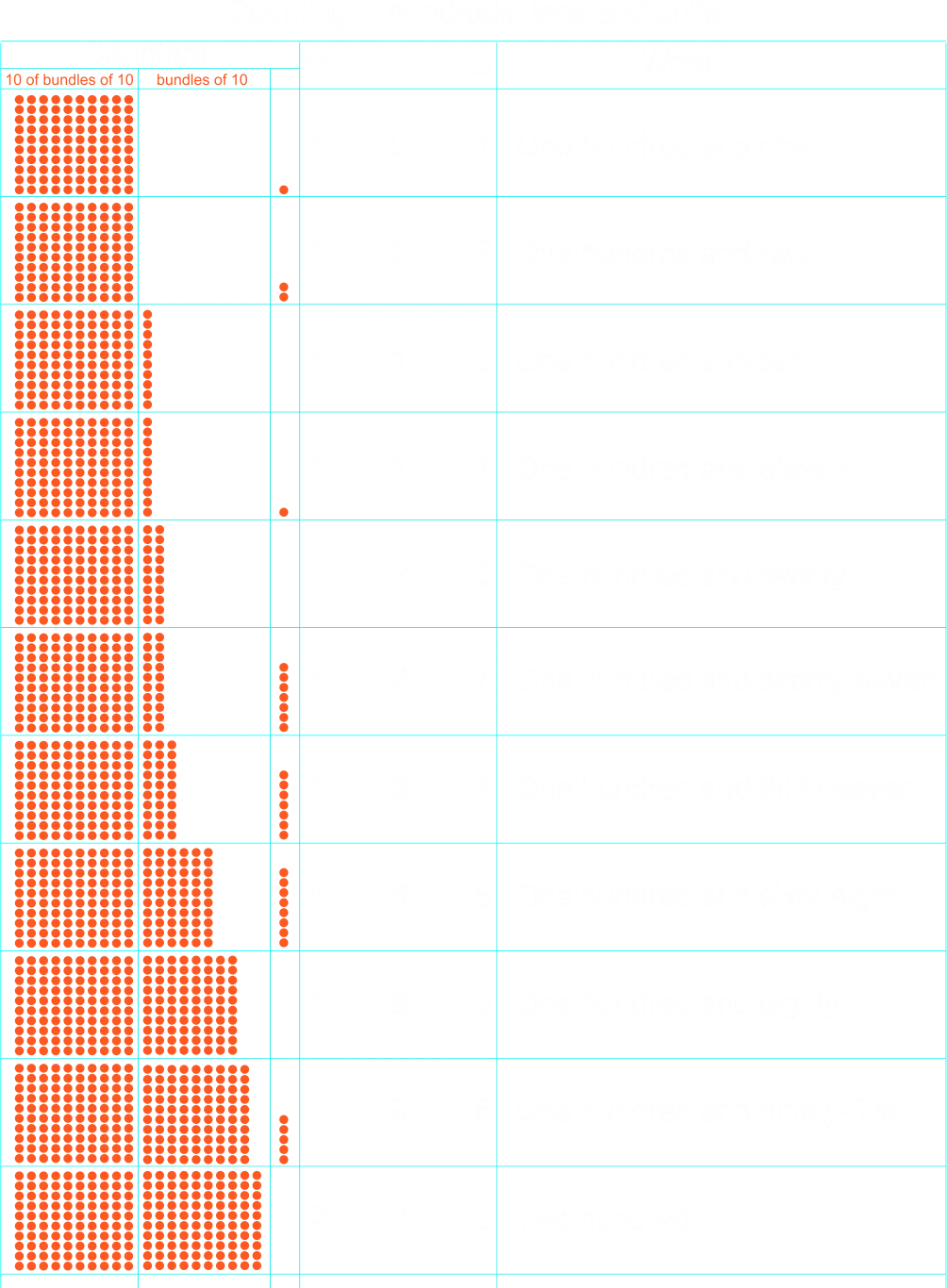
  • Counting up to 200 in tens 2
 Review of past lesson on counting 1-200
ACTIVITY 1
Step 2.
Group Work (5 mins)
Addition of two 3-digit numbers without renaming		 The teacher guides pupils to add two 3-digit numbers without renaming
Tell learners to start adding with the Units, then the Tens and lastly the Hundreds

(Expanded method)
321 + 122
321 = 300 + 20 + 1
122 = 100 + 20 + 2
443 = 400 + 40 + 3 = 443


(Vertical method)
Remind learners to also start adding with the Units, then the Tens and lastly the Hundreds
  • addition 7
 Learners add the following numbers using the expanded method.

1) 193 + 405
193 = 100 + 90 + 3
405 = 400 + 00 + 5
598 = 500 + 90 + 8 = 598

2) 325 + 403
325 = 300 + 20 + 5
403 = 400 + 00 + 3
727 = 700 + 20 + 8 = 728

3) 453 + 130
453 = 400 + 50 + 3
130 = 100 + 30 + 0
583 = 500 + 80 + 3 = 583

4) 631 + 144
631 = 300 + 30 + 1
144 = 100 + 40 + 4
775 = 700 + 70 + 5 = 775

5) 315 + 203
315 = 300 + 10 + 5
203 = 200 + 00 + 3
518 = 500 + 10 + 8 = 581

6) 400 + 513
400 = 400 + 00 + 0
513 = 500 + 10 + 3
913 = 900 + 10 + 3 = 913



Learners add the following numbers using the vertical method
  • addition 8
 Addition of two 3-digit numbers without renaming
ACTIVITY 2
STEP 3
3 mins.
Addition of 2-digit numbers with renaming		 The teacher asks learners that they have learnt that when adding more than 1-digits numbers together they should first and always start with the Unit before adding the Tens and that the Unit can never be 10-99 but 0-9; any single digit numbers.
Now, if after adding the Units digit the result obtained is Tens digit number, what will you do?

The teacher allow learners to brainstorm..., prompt learners to remove (take-away) the bundle of 10 from the Unit and add it to the Tens, and let the Unit that remains to stands allow.
The teacher guides learners to add 36 and 58 together
36 + 58
30 + 6 + 50 + 8
30 + 50 + 6 + 8
80 + 14 (14 = 10 + 4)
80 + 10 + 4
90 + 4 = 94

  • addition 8



The teacher give learners some try.		 The teacher leads the pupils to start addition from the units before proceeding to the tens. Carry the tens from the units and add to the tens column. Add up to give the final answer.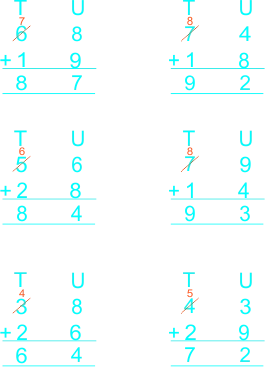

  • addition 10
 Addition of 2-digit numbers with renaming
ACTIVITY 3
Step 4
5 mins.
Mental mathematics		 Finding the ten
The teacher introduces the learners to a mental mathematics trick that will help learners develop their procedural fluency.
Instead of adding two numbers together as they are, the learners are encourage to look at the larger digit to be added to the smaller digit, to think of what figure when added to the larger digit that will make the larger digit 10, such digit should be taken from the smaller digit, and the remainder written down and 1 carried and added to the Tens column if the digit added to the larger figure makes it 10 or above

E.g
1) 68 + 26
2) 25 + 47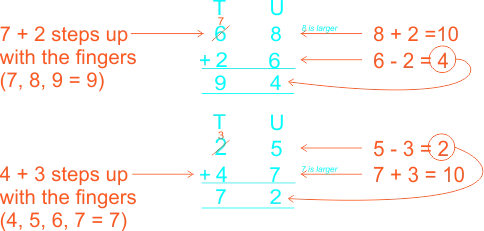
  • addition 11
 Learners use mental mathematics to add the following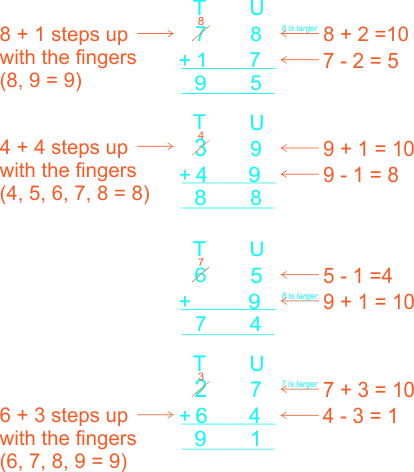
  • addition 12
 Use of mental mathematics to add of 2-digit numbers with renaming
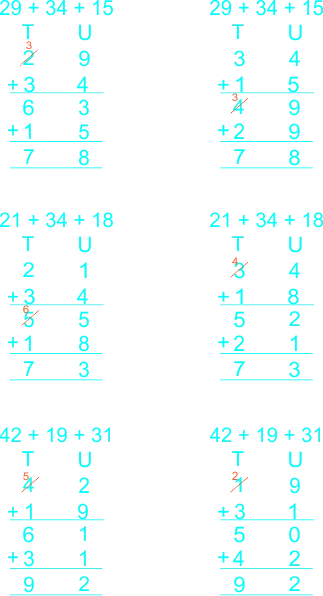
Step 4
5 mins.
Addition of 3 whole numbers taking two at a time		 The teacher tells a story, John has 4 counters, his father gave him 9 more counters while his mother added 8 counters. How many counters have John?

The teacher tells learners to add John's 5 counters to the 9 counters given to him by his father and then add the total to the 8 counters given to him by his mother.
Or, they should add the 9 counters and 8 counters given to John by his parent and the add the total to John's counters.

4 counters + 9 counters + 8 counters
= (4 counters + 9 counters) + 8 counters
= 13 counters + 8 counters
= 21 counters

4 counters + 9 counters + 8 counters
= 4 counters + (9 counters + 8 counters)
= 4 counters + 17 counters
= 21 counters

12 + 4 + 6
= (12 + 4) + 6
= 16 + 6
= 22

12 + 4 + 6
= 12 + (4 + 6)
= 12 + 10
= 22


The teacher guides learners to add the following under learner's activity
 Learners expected answers
1) 5 + 7 + 6
= (5 + 7) + 6
= 12 + 6
= 18

2) 8 + 5 + 7
= (8 + 5) + 7
= 13 + 7
= 20

3) 6 + 7 + 4
= 6 + (7 + 4)
= 6 + 11
= 17

4) 14 + 7 + 13
= 14 + (7 + 13)
= 14 + 20
= 34

5) 5 + 13 + 12
= (5 + 13) + 12
= 18 + 12
= 30

6) 11 + 19 + 6
= (11 + 19) + 6
= 30 + 6
36 		Addition of 3 whole numbers taking two at a time
The teacher tells learners that the same procedure is use in vertical method in addition of 3 whole numbers taking two at a time.
The teacher guides learners to add the following under learner's activity.		 Learners add the following
  • addition 13
Evaluation
Full class session (5 mins)		 Ask the following questions to evaluate the achievement of the set objectives.

1) 4 + 6 + 1 = ____

2) 5 + 7 + 8 = ____

3) 12 + 15 + 13 = ____

4) 27 + 20 + 18 = ____

Use vertical method to add the following
5) 97 + 2
6) 53 + 23
7) 29 + 34
8) 64 + 28
9) 21 + 34 + 13
10) 35 + 22 + 11
11) 52 + 9 + 34
12) 32 19 + 31
13) 44 + 19 + 28
14) 16 + 5 + 39

Word problems
15) A car travels 28 km and then 53 km. Find the total distance covered.

16) Jaye filled a container with a 5 litre bowl and 29 litre bucket. What is the capacity of the container?		 Learners expected response:

1) 4 + 6 + 1
= (4 + 6) + 1
= 10 + 1
= 11

2) 5 + 7 + 8
= 5 + (7 + 8)
= 5 + 15
= 20

3) 12 + 15 + 13
= (12 + 15) + 13
= 27 + 13
= 40

4) 27 + 20 + 18
27 + (20 + 18)
= 27 + 38
= 65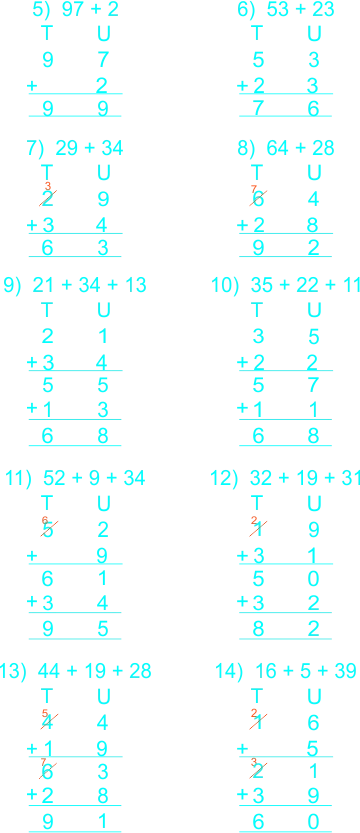

  • addition 14


15) 28 km + 53 km
= 81 km

16) 5 litre + 29 litre
= 34 litre		 Confirming the achievement of the set objectives.
Conclusion, full class session (3 mins) Learners have learnt that
1) Two 2-digits whole numbers can be added by the
– expanded (horizontal) method
– short (direct/vertical) method
for addition without and with renaming.

2) When three whole numbers are added together, the sum or total remains the same irrespective of the two numbers taken first.

3) Real-life activities involving addition can be solved.		 Learners expected answers:
1) Find the sum of thirty-six and fourteen.
Solution
56 + 14 = 70

2) Add forty-nine nuts, twelve nuts and seventeen nuts altogether.
Solution
49 + 12 + 17
= (49 + 12 ) + 17
= 61 + 17
= 78		 Communication: This is developed through answering questions verbally and interpreting the silent signs of teammates during the activities.
ASSIGNMENT The teacher gives learners a take home
1) Find the sum of thirty-six and fourteen.

2) What is the sum of twelve balls and nineteen balls?

3) What is the sum of sixteen oranges and twelve oranges?

4) Find the sum of ten, twenty and nine.

5) Find the sum of three hundred and fifty mangoes and one hundred and twenty mangoes.

6) In a class, there are twenty-three boys and nineteen girls. How many pupils are in the class?

7) In a crate of soft drinks, there are ten bottles of orange flavour and twelve bottles of vanilla flavour.
What is the total number of bottles in the crate of soft drinks?

Use both horizontal and vertical method to add the following
8) 19 + 34 + 26

9) 35 + 14 + 37

10) 29 + 48 + 17

11) 19 + 24 + 23

12) 28 + 19 + 23		 Improving their level of understanding on Fraction




We provide educational resources/materials, curriculum guide, syllabus, scheme of work, lesson note & plan, waec, jamb, O-level & advance level GCE lessons/tutorial classes, on various topics, subjects, career, disciplines & department etc. for all the Class of Learners


Free Will Donation

We know times are tough right now, but if you could donate and support us, be rest assured that your great contributions are immensely appreciated and will be for the progress of our work to help us pay for the server cost, domain renewal, and other maintenance costs of the site. Nothing is too small; nothing is too little.

Account Details

BANK: UNITED BANK FOR AFRICA PLC

ACCOUNT NAME: OFAGBE GODSPOWER GEORGE

ACCOUNT NUMBER: 2250582550

SWIFT CODE: UNAFNGLA

ACCOUNT TYPE: SAVINGS

CURRENCY: DOLLAR (USD) ACCOUNT

ADDRESS: 1. M. Aruna Close, Ughelli, Delta State, Nigeria

PHONE: +234805 5084784, +234803 5586470



BANK: UNITED BANK FOR AFRICA Plc (UBA)

ACCOUNT NAME: OFAGBE GODSPOWER GEORGE

ACCOUNT NUMBER: 2042116266

SORT CODE: 033243371

ACCOUNT TYPE: SAVINGS

CURRENCY: NAIRA ACCOUNT

ADDRESS: 1. M. Aruna Close, Ughelli, Delta State, Nigeria

PHONE: +234805 5084784, +234803 5586470



Your active support gives strength to our Team and inspires to work. Each donated dollar is not only money for us, but it is also the confidence that you really need our project!
AseiClass is a non-profit project that exists at its founders' expense, it will be difficult to achieve our goals without your help.
Please consider making a donation.
Thank you.


AseiClass Team

We provide educational resources/materials, curriculum guide, syllabus, scheme of work, lesson note & plan, waec, jamb, O-level & advance level GCE lessons/tutorial classes, on various topics, subjects, career, disciplines & department etc. for all the Class of Learners

Facts about Teachers

● ● ● Teachers Are Great No Controversy.

● ● ● Teachers are like candles, they burn themselves to light others.

● ● ● Teachers don't teach for the money.

● ● ● Every great mind was once taught by some brilliant teachers.

● ● ● Teachers are the second parents we have.

● ● ● If you can write your name, thank your teacher.

Teaching slogans

● ● ● Until the learner learns the teacher has not taught.

● ● ● I hear and forget, I see and remember, I do and know.

● ● ● The good teacher explains. The superior teacher demonstrates. The great teacher inspires.